Prototype Calculus Question

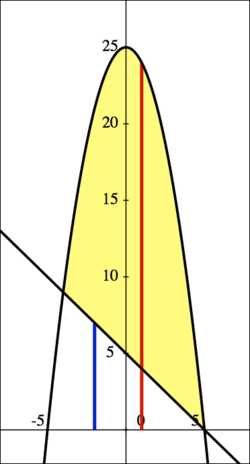
Find the volume of the solid obtained by rotating the area enclosed by $y=5-x$ and $y=25-x^{2}$
around the x-axis.

Foundations
Choose either shell or washer method.
Find the appropriate radii.
Determine the bounds of integration by finding when both functions have the same y value.
Using the determined values, set up and solve the integral.

Solution:

Step 1:
Since we are rotating around the x-axis, the washer method would utilize tall rectangles with dx as their width. This seems like a reasonable choice, as these rectangles would be trapped between our two functions, allowing us to solve a single integral.

Step 2:
Since our rectangles will be trapped between the two functions, and will be rotated around the x-axis (where $y=0$,) we find
the inner radius is $r=5-x$, represented by the blue line, while
the outer radius is $R=25-x^{2}$, represented by the red line.

Step 3:
We must set the two functions equal, and solve. More to follow...