Difference between revisions of "022 Exam 2 Sample B, Problem 10"
Jump to navigation
Jump to search
| (4 intermediate revisions by the same user not shown) | |||
| Line 7: | Line 7: | ||
!Foundations: | !Foundations: | ||
|- | |- | ||
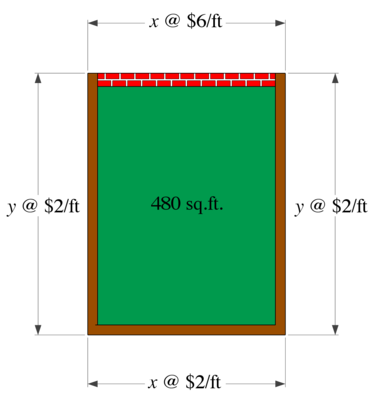
| − | |As with all geometric word problems, it helps to start with a picture. Using the variables <math style="vertical-align: 0%">x</math> and <math style="vertical-align: -20%">y</math> as shown in the image, we need to remember the | + | |As with all geometric word problems, it helps to start with a picture. Using the variables <math style="vertical-align: 0%">x</math> and <math style="vertical-align: -20%">y</math> as shown in the image, we need to remember the equation for the area of a rectangle: |
|- | |- | ||
| | | | ||
| Line 25: | Line 25: | ||
!Step 1: | !Step 1: | ||
|- | |- | ||
| − | |'''Express one variable in terms of the other:''' Since we know that the area is 480 square feet and <math style="vertical-align: - | + | |'''Express one variable in terms of the other:''' Since we know that the area is 480 square feet and <math style="vertical-align: -15%">A\,=\,xy</math>, we can solve for <math style="vertical-align: -15%">y</math> in terms of <math style="vertical-align: 0%">x</math>. Since <math style="vertical-align: -17%">480\,=\,xy</math>, we find that <math style="vertical-align: -20%">y=480/x</math>. |
|} | |} | ||
| Line 31: | Line 31: | ||
!Step 2: | !Step 2: | ||
|- | |- | ||
| − | |'''Find an expression for cost in terms of one variable:''' Now, we can use the substitution from | + | |'''Find an expression for cost in terms of one variable:''' Now, we can use the substitution from step 1 to find |
|- | |- | ||
| | | | ||
| Line 45: | Line 45: | ||
::<math>C'(x)\,=\,8-\frac{1920}{x^2}\,=\,8\left(1-\frac{240}{x^2}\right).</math> | ::<math>C'(x)\,=\,8-\frac{1920}{x^2}\,=\,8\left(1-\frac{240}{x^2}\right).</math> | ||
|- | |- | ||
| − | |This derivative is zero precisely when <math style="vertical-align: - | + | |This derivative is zero precisely when <math style="vertical-align: -8%">x=4\sqrt{15}</math>, which occurs when <math style="vertical-align: -18%">y=8\sqrt{15}</math>, and these are the values that will minimize cost. Also, don't forget the units - feet! |
|} | |} | ||
Latest revision as of 16:35, 17 May 2015
Use calculus to set up and solve the word problem: A fence is to be built to enclose a rectangular region of 480 square feet. The fencing material along three sides cost $2 per foot. The fencing material along the 4th side costs $6 per foot. Find the most economical dimensions of the region (that is, minimize the cost).
| Foundations: |
|---|
| As with all geometric word problems, it helps to start with a picture. Using the variables and as shown in the image, we need to remember the equation for the area of a rectangle: |
|
|
| However, we need to construct a new function to describe cost: |
|
|
| Since we want to minimize cost, we will have to rewrite it as a function of a single variable, and then find when the first derivative is zero. From this, we will find the dimensions which provide the minimum cost. |
Solution:
| Step 1: |
|---|
| Express one variable in terms of the other: Since we know that the area is 480 square feet and , we can solve for in terms of . Since , we find that . |
| Step 2: |
|---|
| Find an expression for cost in terms of one variable: Now, we can use the substitution from step 1 to find |
|
|
| Step 3: |
|---|
| Find the derivative and its roots: We can apply the power rule term-by-term to find |
|
|
| This derivative is zero precisely when , which occurs when , and these are the values that will minimize cost. Also, don't forget the units - feet! |
| Final Answer: |
|---|
| The cost is minimized when the dimensions are feet by feet. Note that the side with the most expensive fencing is the shorter one. |