Difference between revisions of "004 Sample Final A, Problem 4"

From Math Wiki
Jump to navigation Jump to search
(Created page with "<span class="exam"> Graph the system of inequalities. <math>y > 2x - 3 \qquad y \le 4-x^2</math> {| class="mw-collapsible mw-collapsed" style = "text-align:left;" ! Foundation...")
 
Line 1: Line 1:
 
<span class="exam"> Graph the system of inequalities. <math>y > 2x - 3 \qquad y \le 4-x^2</math>
 
<span class="exam"> Graph the system of inequalities. <math>y > 2x - 3 \qquad y \le 4-x^2</math>
 +
Solution:
 +
 
{| class="mw-collapsible mw-collapsed" style = "text-align:left;"
 
{| class="mw-collapsible mw-collapsed" style = "text-align:left;"
−
! Foundations
+
! Step 1: &nbsp;
−
|-
 
−
|How do we graph <math>y>x</math>?
 
−
|-
 
−
|Answer:
 
−
|-
 
−
|We replace the inequality <math>></math> with <math>=</math>. Now, we have the equation of a line.
 
 
|-
 
|-
−
|Since the sign was <math>></math> and not <math>\geq </math>, the line is dashed. Now, since <math>y>x</math>,
+
|First we replace the inequalities with equality. So <math>y = \vert x\vert + 1</math>, and <math>x^2 + y^2 = 9</math>.
 
|-
 
|-
−
|we shade in the area to the left of the line.  
+
|Now we graph both functions.
 
|}
 
|}
  
−
 
+
{| class="mw-collapsible mw-collapsed" style = "text-align:left;"
−
Solution:
+
! Step 2: &nbsp;
−
 
 
−
{| class = "mw-collapsible mw-collapsed" style = "text-align:left;"
 
−
! Step 1:
 
 
|-
 
|-
−
|
+
|Now that we have graphed both functions we need to know which region to shade with respect to each graph.
 
|-
 
|-
−
|
+
|To do this we pick a point an equation and a point not on the graph of that equation. We then check if the
−
|}
 
−
 
 
−
{|class = "mw-collapsible mw-collapsed" style = "text-align:left;"
 
−
! Step 2:
 
 
|-
 
|-
−
|
+
|point satisfies the inequality or not. For both equations we will pick the origin.
−
|}
 
−
 
 
−
{|class = "mw-collapsible mw-collapsed" style = "text-align:left;"
 
−
! Step 3:
 
 
|-
 
|-
−
|
+
|<math>y < \vert x\vert + 1:</math> Plugging in the origin we get, <math>0 < \vert 0\vert + 1 = 1</math>. Since the inequality is satisfied shade the side of
 
|-
 
|-
−
|
+
|<math>y < \vert x\vert + 1</math> that includes the origin. We make the graph of <math>y < \vert x\vert + 1</math>, since the inequality is strict.
 
|-
 
|-
−
|
+
|<math>x^2 + y^2 \le 9:</math> <math>(0)^2 +(0)^2 = 0 \le 9</math>. Once again the inequality is satisfied. So we shade the inside of the circle.
 
|-
 
|-
−
|
+
|We also shade the boundary of the circle since the inequality is <math>\le</math>
 
|}
 
|}
  
−
{|class = "mw-collapsible mw-collapsed" style = "text-align:left;"
+
{| class="mw-collapsible mw-collapsed" style = "text-align:left;"
−
! Step 4:
+
! Final Answer: &nbsp;
 
|-
 
|-
−
|
+
|The final solution is the portion of the graph that below <math>y = \vert x\vert + 1</math> and inside <math> x^2 + y^2 = 9</math>
 
|-
 
|-
−
|
+
|The region we are referring to is shaded both blue and red.
 
|-
 
|-
−
|
+
|[[File:8A_Final_5.png]]
 
|}
 
|}
  
−
{|class = "mw-collapsible mw-collapsed" style = "text-align:left;"
 
−
! Final Answer:
 
−
|-
 
−
|
 
−
|}
 
  
 
[[004 Sample Final A|<u>'''Return to Sample Exam</u>''']]
 
[[004 Sample Final A|<u>'''Return to Sample Exam</u>''']]

Revision as of 09:01, 2 June 2015

Graph the system of inequalities. Solution:

Step 1:  
First we replace the inequalities with equality. So Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y = \vert x\vert + 1} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x^2 + y^2 = 9} .
Now we graph both functions.
Step 2:  
Now that we have graphed both functions we need to know which region to shade with respect to each graph.
To do this we pick a point an equation and a point not on the graph of that equation. We then check if the
point satisfies the inequality or not. For both equations we will pick the origin.
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y < \vert x\vert + 1:} Plugging in the origin we get, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0 < \vert 0\vert + 1 = 1} . Since the inequality is satisfied shade the side of
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y < \vert x\vert + 1} that includes the origin. We make the graph of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y < \vert x\vert + 1} , since the inequality is strict.
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x^2 + y^2 \le 9:} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (0)^2 +(0)^2 = 0 \le 9} . Once again the inequality is satisfied. So we shade the inside of the circle.
We also shade the boundary of the circle since the inequality is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \le}
Final Answer:  
The final solution is the portion of the graph that below Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y = \vert x\vert + 1} and inside Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x^2 + y^2 = 9}
The region we are referring to is shaded both blue and red.
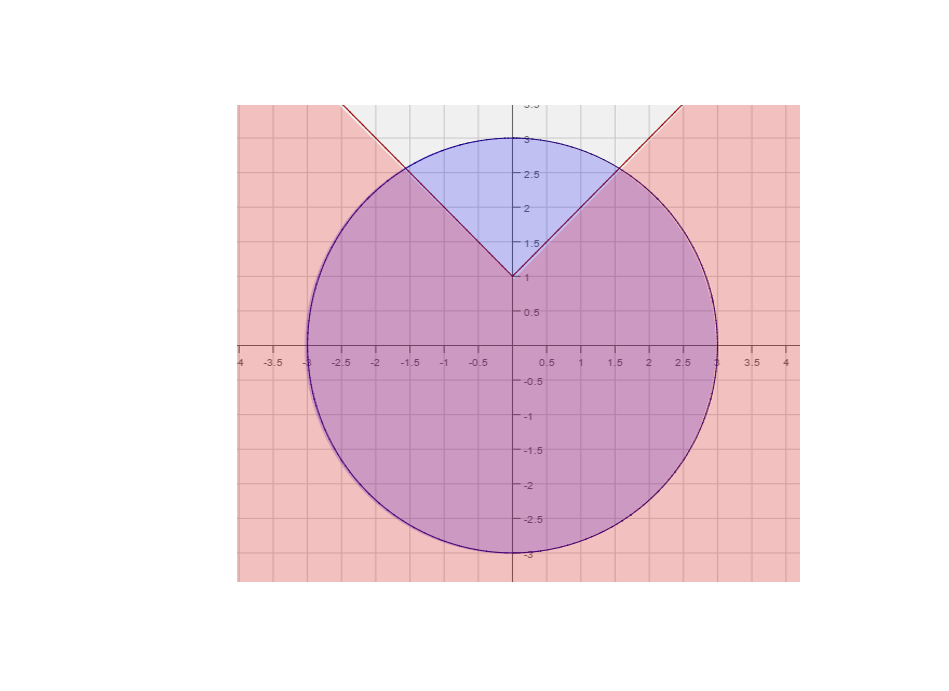
8A Final 5.png


Return to Sample Exam

Retrieved from "https://wiki.math.ucr.edu/index.php?title=004_Sample_Final_A,_Problem_4&oldid=829"