Difference between revisions of "009B Sample Midterm 3, Problem 1"
Jump to navigation
Jump to search
(Created page with "<span class="exam">Divide the interval <math style="vertical-align: -5px">[0,\pi]</math> into four subintervals of equal length <math>\frac{\pi}{4}</math> and compute the righ...") |
|||
| Line 1: | Line 1: | ||
| + | [[File:9B_SM3_1_GP.png|right|375px]] | ||
| + | |||
<span class="exam">Divide the interval <math style="vertical-align: -5px">[0,\pi]</math> into four subintervals of equal length <math>\frac{\pi}{4}</math> and compute the right-endpoint Riemann sum of <math style="vertical-align: -5px">y=\sin (x).</math> | <span class="exam">Divide the interval <math style="vertical-align: -5px">[0,\pi]</math> into four subintervals of equal length <math>\frac{\pi}{4}</math> and compute the right-endpoint Riemann sum of <math style="vertical-align: -5px">y=\sin (x).</math> | ||
Revision as of 14:27, 12 May 2016
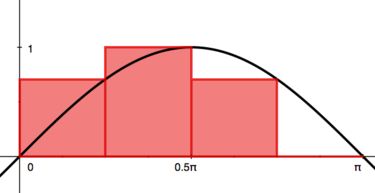
Divide the interval into four subintervals of equal length and compute the right-endpoint Riemann sum of
| Foundations: |
|---|
| Recall: |
|
|
Solution:
| Step 1: |
|---|
| Let Each interval has length |
| So, the right-endpoint Riemann sum of on the interval is |
|
|
| Step 2: |
|---|
| Thus, the right-endpoint Riemann sum is |
|
|
| Final Answer: |
|---|
![{\displaystyle [0,\pi ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3e2a912eda6ef1afe46a81b518fe9da64a832751)







