Difference between revisions of "008A Sample Final A, Question 6"
Jump to navigation
Jump to search
| Line 2: | Line 2: | ||
{| class="mw-collapsible mw-collapsed" style = "text-align:left;" | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| − | !Foundations | + | !Foundations: |
|- | |- | ||
|1) What type of function is this? | |1) What type of function is this? | ||
| Line 18: | Line 18: | ||
{| class="mw-collapsible mw-collapsed" style = "text-align:left;" | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| − | ! Step 1: | + | ! Step 1: |
|- | |- | ||
|We start by dividing both sides by 36. This yields <math>\frac{4x^2}{36} + \frac{9(y + 1)^2}{36} = \frac{x^2}{9} + \frac{(y+1)^2}{4} = 1</math>. | |We start by dividing both sides by 36. This yields <math>\frac{4x^2}{36} + \frac{9(y + 1)^2}{36} = \frac{x^2}{9} + \frac{(y+1)^2}{4} = 1</math>. | ||
| Line 24: | Line 24: | ||
{| class="mw-collapsible mw-collapsed" style = "text-align:left;" | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| − | ! Step 2: | + | ! Step 2: |
|- | |- | ||
|Now that we have the equation that looks like an ellipse, we can read off the center of the ellipse, (0, -1). | |Now that we have the equation that looks like an ellipse, we can read off the center of the ellipse, (0, -1). | ||
| Line 34: | Line 34: | ||
{| class="mw-collapsible mw-collapsed" style = "text-align:left;" | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| − | ! Final Answer: | + | ! Final Answer: |
|- | |- | ||
|The four vertices are: <math>(-3, -1), (3, -1), (0, 1) \text{ and } (0, -3)</math> | |The four vertices are: <math>(-3, -1), (3, -1), (0, 1) \text{ and } (0, -3)</math> | ||
Latest revision as of 22:52, 25 May 2015
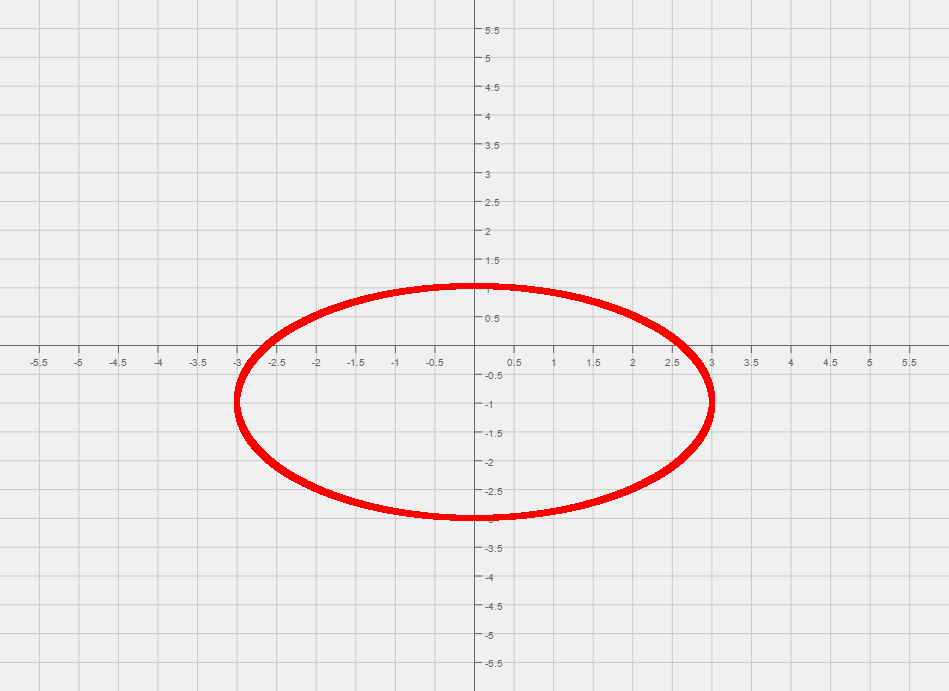
Question: Sketch . Give coordinates of each of the 4 vertices of the graph.
| Foundations: |
|---|
| 1) What type of function is this? |
| 2) What can you say about the orientation of the graph? |
| Answer: |
| 1) Since both x and y are squared it must be a hyperbola or an ellipse. We can conclude that the graph is an ellipse since both and have the same sign, positive. |
| 2) Since the coefficient of the term is smaller, when we divide both sides by 36 the X-axis will be the major axis. |
Solution:
| Step 1: |
|---|
| We start by dividing both sides by 36. This yields . |
| Step 2: |
|---|
| Now that we have the equation that looks like an ellipse, we can read off the center of the ellipse, (0, -1). |
| From the center mark the two points that are 3 units left, and three units right of the center. |
| Then mark the two points that are 2 units up, and two units down from the center. |
| Final Answer: |
|---|
| The four vertices are: |
|




