Difference between revisions of "005 Sample Final A, Question 16"
Jump to navigation
Jump to search
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -x^2+4y^2-2x-16y+11=0}
| Line 10: | Line 10: | ||
|Answer: | |Answer: | ||
|- | |- | ||
| − | |1) Since both x and y are squared it must be a hyperbola or an ellipse. We can conclude that the graph is | + | |1) Since both x and y are squared it must be a hyperbola or an ellipse. We can conclude that the graph is a hyperbola since <math>x^2</math> and <math>y^2</math> have the different signs, one negative and one positive. |
|- | |- | ||
| − | |2) Since | + | |2) Since the <math>y^2</math> is positive, the hyperbola opens up and down. |
|} | |} | ||
| Line 20: | Line 20: | ||
! Step 1: | ! Step 1: | ||
|- | |- | ||
| − | |We start by | + | |We start by completing the square twice, once for x and once for y. After completing the squares we end up with <math>-(x + 1)^2 +4(y - 2)^2 = 4</math> |
| + | |- | ||
| + | |'''Common Mistake:''' When completing the square we will end up adding numbers inside of parenthesis. So make sure you add the correct value to this other side. In this case we add -1, and 16 for completing the square with respect to x and y, respectively. | ||
|} | |} | ||
Revision as of 10:27, 2 June 2015
Question Graph the following,
| Foundations: |
|---|
| 1) What type of function is this? |
| 2) What can you say about the orientation of the graph? |
| Answer: |
| 1) Since both x and y are squared it must be a hyperbola or an ellipse. We can conclude that the graph is a hyperbola since Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x^2} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y^2} have the different signs, one negative and one positive. |
| 2) Since the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y^2} is positive, the hyperbola opens up and down. |
Solution:
| Step 1: |
|---|
| We start by completing the square twice, once for x and once for y. After completing the squares we end up with Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -(x + 1)^2 +4(y - 2)^2 = 4} |
| Common Mistake: When completing the square we will end up adding numbers inside of parenthesis. So make sure you add the correct value to this other side. In this case we add -1, and 16 for completing the square with respect to x and y, respectively. |
| Step 2: |
|---|
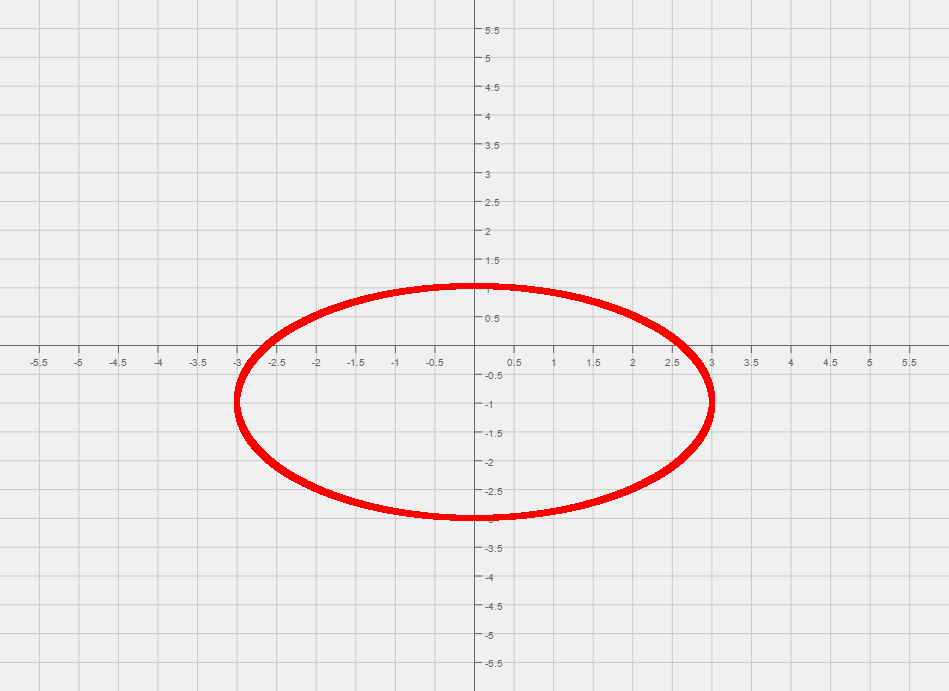
| Now that we have the equation that looks like an ellipse, we can read off the center of the ellipse, (0, -1). |
| From the center mark the two points that are 3 units left, and three units right of the center. |
| Then mark the two points that are 2 units up, and two units down from the center. |
| Final Answer: |
|---|
| The four vertices are: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (-3, -1), (3, -1), (0, 1) \text{ and } (0, -3)} |
|