Difference between revisions of "009A Sample Final A, Problem 7"
Jump to navigation
Jump to search
(Created page with "<br> <span style="font-size:135%"><font face=Times Roman> 7. A farmer wishes to make 4 identical rectangular pens, each with 500 sq. ft. of area. What dimensions for each pen...") |
m |
||
| Line 1: | Line 1: | ||
| + | [[File:009A_SF_A_7_PensDim.png|right|200px]] | ||
<br> | <br> | ||
<span style="font-size:135%"><font face=Times Roman> 7. A farmer wishes to make 4 identical rectangular pens, each with | <span style="font-size:135%"><font face=Times Roman> 7. A farmer wishes to make 4 identical rectangular pens, each with | ||
| Line 12: | Line 13: | ||
{| class="mw-collapsible mw-collapsed" style = "text-align:left;" | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| − | ! | + | !Step 1: |
| + | |- | ||
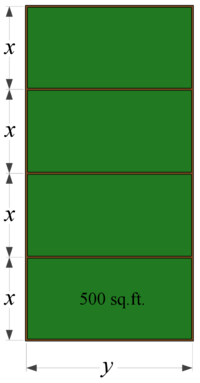
| + | |'''Declare Variables:''' We are attempting to find the dimensions of a single pen, such that we use as little fencing as possible for all four pens. Let's use ''x'' and ''y'' as indicated in the image, and simply call the length of fencing required ''L''. | ||
| + | |} | ||
| + | |||
| + | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| + | !Step 2: | ||
| + | |- | ||
| + | |'''Form the Equations:''' Notice that we need fencing between each of the pens (think "lion-antelope-lion-antelope" if this isn't clear). We require 2 pieces of length ''x'' for each pen, and a total of 5 pieces of length ''y''. Together, we need a total length of <math style="vertical-align: -20%;">L = 8x + 5y. </math> | ||
| + | |- | ||
| + | |On the other hand, we know that each pen has a fixed area of 500 square feet. Thus, we also know that ''xy'' = 500. | ||
| + | |} | ||
| + | |||
| + | {| class="mw-collapsible mw-collapsed" style = "text-align:left;" | ||
| + | !Step 3: | ||
| + | |- | ||
| + | |'''Optimize:''' Since ''xy'' = 500, we also know ''y'' = 500/''x''. Plugging this into our equation for length, we have | ||
| + | |- | ||
| + | | <math>L(x) = 8x + 5\cdot \frac{500}{x} = 8x + \frac{2500}{x}.</math> | ||
| + | |- | ||
| + | |We now take the derivative to find | ||
| + | |- | ||
| + | | <math>L'(x)=8-\frac{2500}{x^2}= \frac{8x^2-2500}{x^2}.</math> | ||
| + | |- | ||
| + | |The denominator can never be zero, and if we set the numerator to zero we find | ||
| + | |- | ||
| + | | <math>x=\pm\sqrt{\frac{2500}{8}} = \pm\frac{50}{2\sqrt{2}} =\pm\frac{\,\,25}{\sqrt{2}}.</math> | ||
| + | |- | ||
| + | |Of course, we can't have negative fencing lengths, so we can ignore the negative root. Finally, we use the area relation to find | ||
| + | |- | ||
| + | | <math>y =\frac{500}{25\sqrt{2}} = \frac{500\sqrt{2}}{25} = 20\sqrt{2}. </math> | ||
| + | |- | ||
| + | |Thus, the least amount of fencing is used when we size our 500 sq. ft. pens as 20√<span style="text-decoration:overline">2</span> feet by 25/√<span style="text-decoration:overline">2</span> feet. | ||
| + | |} | ||
| + | |||
[[009A_Sample_Final_A|'''<u>Return to Sample Exam</u>''']] | [[009A_Sample_Final_A|'''<u>Return to Sample Exam</u>''']] | ||
Revision as of 11:56, 24 March 2015
7. A farmer wishes to make 4 identical rectangular pens, each with
500 sq. ft. of area. What dimensions for each pen will use the least
amount of total fencing?
| Foundations: |
|---|
| As a word problem, we must begin by assigning variables in order to construct useful equation(s). As an optimization problem, we will be taking a derivative of one of our equations in order to find an extreme point. |
Solution:
| Step 1: |
|---|
| Declare Variables: We are attempting to find the dimensions of a single pen, such that we use as little fencing as possible for all four pens. Let's use x and y as indicated in the image, and simply call the length of fencing required L. |
| Step 2: |
|---|
| Form the Equations: Notice that we need fencing between each of the pens (think "lion-antelope-lion-antelope" if this isn't clear). We require 2 pieces of length x for each pen, and a total of 5 pieces of length y. Together, we need a total length of |
| On the other hand, we know that each pen has a fixed area of 500 square feet. Thus, we also know that xy = 500. |
| Step 3: |
|---|
| Optimize: Since xy = 500, we also know y = 500/x. Plugging this into our equation for length, we have |
| We now take the derivative to find |
| The denominator can never be zero, and if we set the numerator to zero we find |
| Of course, we can't have negative fencing lengths, so we can ignore the negative root. Finally, we use the area relation to find |
| Thus, the least amount of fencing is used when we size our 500 sq. ft. pens as 20√2 feet by 25/√2 feet. |