Difference between revisions of "009B Sample Final 1, Problem 5"

From Math Wiki
Jump to navigation Jump to search
Line 3: Line 3:
 
::::::<span class="exam"> <math style="vertical-align: 0px">x=0</math>, <math style="vertical-align: -4px">y=e^x</math>, and <math style="vertical-align: -4px">y=ex</math>.
 
::::::<span class="exam"> <math style="vertical-align: 0px">x=0</math>, <math style="vertical-align: -4px">y=e^x</math>, and <math style="vertical-align: -4px">y=ex</math>.
  
−
<span class="exam">a) Sketch the region bounded by the given three functions. Find the intersection point of the two functions:  
+
::<span class="exam">a) Sketch the region bounded by the given three functions. Find the intersection point of the two functions:  
  
−
:<span class="exam"><math style="vertical-align: -4px">y=e^x</math> and <math style="vertical-align: -4px">y=ex</math>. (There is only one.)
+
::::::<span class="exam"><math style="vertical-align: -4px">y=e^x</math> and <math style="vertical-align: -4px">y=ex</math>. (There is only one.)
  
−
<span class="exam">b) Set up the integral for the volume of the solid.
+
::<span class="exam">b) Set up the integral for the volume of the solid.
  
−
<span class="exam">c) Find the volume of the solid by computing the integral.
+
::<span class="exam">c) Find the volume of the solid by computing the integral.
  
 
{| class="mw-collapsible mw-collapsed" style = "text-align:left;"
 
{| class="mw-collapsible mw-collapsed" style = "text-align:left;"
Line 16: Line 16:
 
|Recall:
 
|Recall:
 
|-
 
|-
−
|'''1.''' You can find the intersection points of two functions, say <math style="vertical-align: -5px">f(x),g(x),</math>
+
|
 +
::'''1.''' You can find the intersection points of two functions, say <math style="vertical-align: -5px">f(x),g(x),</math>
 
|-
 
|-
 
|
 
|
−
::by setting <math style="vertical-align: -5px">f(x)=g(x)</math> and solving for <math style="vertical-align: 0px">x</math>.
+
:::by setting <math style="vertical-align: -5px">f(x)=g(x)</math> and solving for <math style="vertical-align: 0px">x.</math>
 
|-
 
|-
−
|'''2.''' The volume of a solid obtained by rotating an area around the <math style="vertical-align: -4px">y</math>-axis using cylindrical shells is given by   
+
|
 +
::'''2.''' The volume of a solid obtained by rotating an area around the <math style="vertical-align: -4px">y</math>-axis using cylindrical shells is given by   
 
|-
 
|-
 
|
 
|
−
::<math style="vertical-align: -13px">\int 2\pi rh~dx,</math> where <math style="vertical-align: 0px">r</math> is the radius of the shells and <math style="vertical-align: 0px">h</math> is the height of the shells.
+
:::<math style="vertical-align: -13px">\int 2\pi rh~dx,</math> where <math style="vertical-align: 0px">r</math> is the radius of the shells and <math style="vertical-align: 0px">h</math> is the height of the shells.
 
|}
 
|}
  
Line 44: Line 46:
 
!Step 2: &nbsp;
 
!Step 2: &nbsp;
 
|-
 
|-
−
|Setting the equations equal, we have <math style="vertical-align: 0px">e^x=ex</math>.
+
|Setting the equations equal, we have <math style="vertical-align: 0px">e^x=ex.</math>
 
|-
 
|-
−
|We get one intersection point, which is <math style="vertical-align: -4px">(1,e)</math>.
+
|We get one intersection point, which is <math style="vertical-align: -4px">(1,e).</math>
 
|-
 
|-
 
|This intersection point can be seen in the graph shown in Step 1.
 
|This intersection point can be seen in the graph shown in Step 1.
Line 56: Line 58:
 
!Step 1: &nbsp;  
 
!Step 1: &nbsp;  
 
|-
 
|-
−
|We proceed using cylindrical shells. The radius of the shells is given by <math style="vertical-align: 0px">r=x</math>.
+
|We proceed using cylindrical shells. The radius of the shells is given by <math style="vertical-align: 0px">r=x.</math>
 +
|-
 +
|The height of the shells is given by
 
|-
 
|-
−
|The height of the shells is given by <math style="vertical-align: 0px">h=e^x-ex</math>.
+
|
 +
::<math style="vertical-align: 0px">h=e^x-ex.</math>
 
|}
 
|}
  
Line 86: Line 91:
 
|For the first integral, we need to use integration by parts.  
 
|For the first integral, we need to use integration by parts.  
 
|-
 
|-
−
|Let <math style="vertical-align: 0px">u=x</math> and <math style="vertical-align: 0px">dv=e^xdx</math>. Then, <math style="vertical-align: 0px">du=dx</math> and <math style="vertical-align: 0px">v=e^x</math>.
+
|Let <math style="vertical-align: 0px">u=x</math> and <math style="vertical-align: 0px">dv=e^xdx.</math> Then, <math style="vertical-align: 0px">du=dx</math> and <math style="vertical-align: 0px">v=e^x.</math>  
 
|-
 
|-
 
|So, the integral becomes
 
|So, the integral becomes

Revision as of 12:07, 18 April 2016

Consider the solid obtained by rotating the area bounded by the following three functions about the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y} -axis:

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=0} , Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=e^x} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=ex} .
a) Sketch the region bounded by the given three functions. Find the intersection point of the two functions:
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=e^x} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=ex} . (There is only one.)
b) Set up the integral for the volume of the solid.
c) Find the volume of the solid by computing the integral.
Foundations:  
Recall:
1. You can find the intersection points of two functions, say Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(x),g(x),}
by setting Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(x)=g(x)} and solving for Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x.}
2. The volume of a solid obtained by rotating an area around the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y} -axis using cylindrical shells is given by
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int 2\pi rh~dx,} where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r} is the radius of the shells and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h} is the height of the shells.

Solution:

(a)

Step 1:  
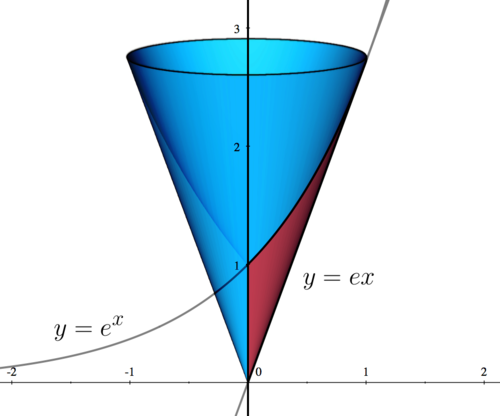
First, we sketch the region bounded by the three functions. The region is shown in red, while the revolved solid is shown in blue.
 
9BF1 5 GP.png
Step 2:  
Setting the equations equal, we have Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e^x=ex.}
We get one intersection point, which is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (1,e).}
This intersection point can be seen in the graph shown in Step 1.

(b)

Step 1:  
We proceed using cylindrical shells. The radius of the shells is given by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r=x.}
The height of the shells is given by
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h=e^x-ex.}
Step 2:  
So, the volume of the solid is
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int 2\pi rh\,dx\,=\,\int_0^1 2\pi x(e^x-ex)\,dx.}

(c)

Step 1:  
We need to integrate
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_0^1 2\pi x(e^x-ex)\,dx\,=\,2\pi\int_0^1 xe^x\,dx-2\pi\int_0^1ex^2\,dx.}
Step 2:  
For the first integral, we need to use integration by parts.
Let Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u=x} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dv=e^xdx.} Then, and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle v=e^x.}
So, the integral becomes
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} \displaystyle{\int_0^1 2\pi x(e^x-ex)~dx} & = & \displaystyle{2\pi\bigg(xe^x\bigg|_0^1 -\int_0^1 e^xdx\bigg)-\frac{2\pi ex^3}{3}\bigg|_0^1}\\ &&\\ & = & \displaystyle{2\pi\bigg(xe^x-e^x\bigg)\bigg|_0^1-\frac{2\pi e}{3}}\\ &&\\ & = & \displaystyle{2\pi(e-e-(-1))-\frac{2\pi e}{3}}\\ &&\\ & = & \displaystyle{2\pi-\frac{2\pi e}{3}}.\\ \end{array}}
Final Answer:  
(a)   (See Step 1 for the graph)
(b)  Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_0^1 2\pi x(e^x-ex)~dx}
(c)  Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2\pi-\frac{2\pi e}{3}}

Return to Sample Exam

Retrieved from "https://wiki.math.ucr.edu/index.php?title=009B_Sample_Final_1,_Problem_5&oldid=1330"